

PROBLEM 2 : Use the Intermediate Value Theorem to prove that the equation $ e^x = 4-x^3 $ is solvable on the interval.Ĭlick HERE to see a detailed solution to problem 1.
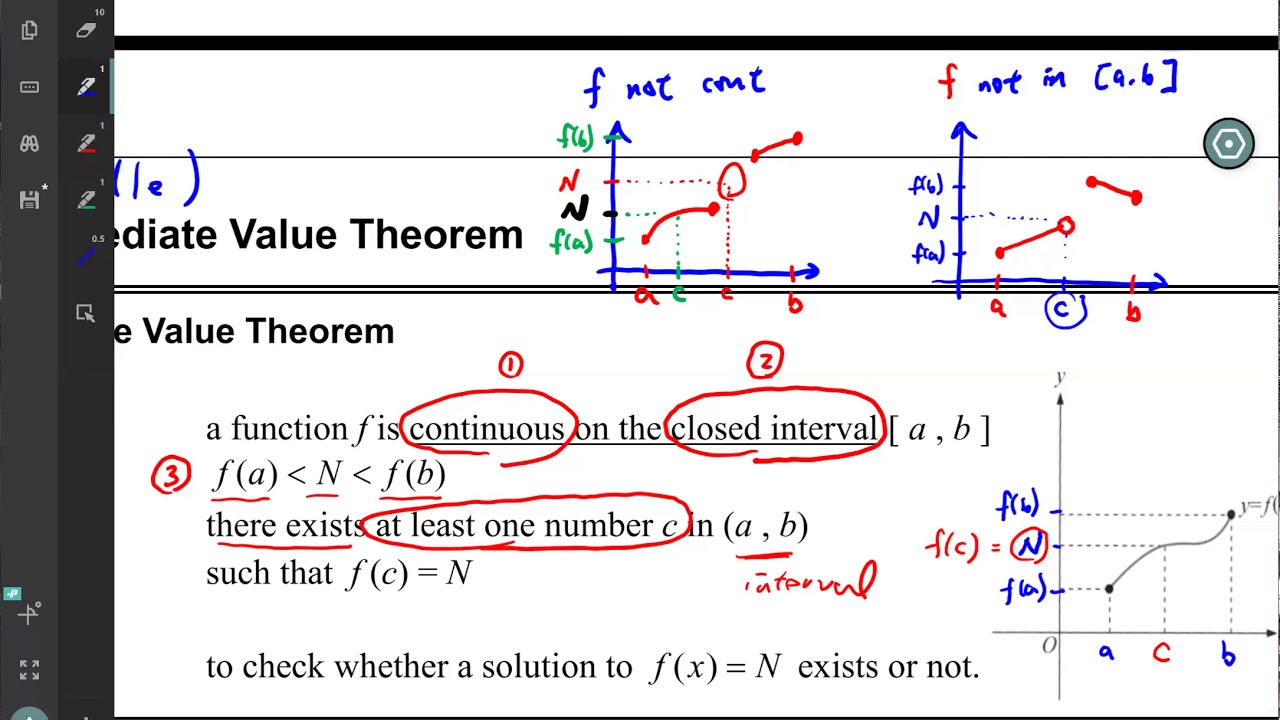
PROBLEM 1 : Use the Intermediate Value Theorem to prove that the equation $ 3x^5-4x^2=3 $ is solvable on the interval.In the list of Differentials Problems which follows, most problems are average and a few are somewhat challenging. Now invoke the conclusion of the Intermediate Value Theorem. Establish that $m$ is between $ f(a) $ and $ f(b) $. Here is a summary of how I will use the Intermediate Value Theorem in the problems that follow. It should be noted that Intermediate Value Theorem guarantees the existence of a solution, but not what the solution is. This result is formally accredited to Berard Bolzano and is calledīolzano's Theorem. Then the conditions $ f(a)0 $ would lead to the conclusion that the equation $f(x)=0$ is solvable for $x$, i.e., $f(c)=0$. While the result certainly seems intuitively obvious, the formal proof of the Intermediate Value Theorem is quite sophisticated and is beyond the experience of most first-year calculus students.įor a simple illustration of the this theorem, assume that a function $f$ is a continuous and $m=0$. Then there is at least one number $c$ ($x$-value) in the interval $$ which satifies Assume that $m$ is a number ($y$-value) between $f(a)$ and $f(b)$. INTERMEDIATE VALUE THEOREM: Let $f$ be a continuous function on the closed interval $ $. All functions are assumed to be real-valued. The formal statement of this theorem together with an illustration of the theorem follow. (Newton's Method could be used to determine a good ESTIMATE for these solutions.) Note that this theorem will be used to prove the EXISTENCE of solutions, but will not actually solve the equations. Generally speaking, the Intermediate Value Theorem applies to continuous functions and is used to prove that equations, both algebraic and transcendental, are solvable. History of this theorem begins in the 1500's and is eventually based on the academic work of Mathematicians Bernard Bolzano, Augustin-Louis Cauchy, Joseph-Louis Lagrange, and Simon Stevin. The Intermediate Value Theorem is one of the most important theorems in Introductory Calculus, and it forms the basis for proofs of many results in subsequent and advanced Mathematics courses. Solving Intermediate Value Theorem Problems


 0 kommentar(er)
0 kommentar(er)
